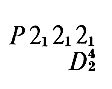
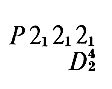
In the previous chapter we have shown that the combination of all available symmetry operations (point group operations plus glides and screws) with the Bravais translations leads to exactly 230 combinations, the 230 Space Groups. The International Tables (IT) list those by symbol and number, together with symmetry operators, origins, reflection conditions, and space group projection diagrams. We will go into more detail of space groups now and we will use an interactive web program to learn more about the space groups.
The commonly used space group symbols are the short Hermann-Mauguin symbols, consisting of a Bravais symbol followed by the symmetry operators generating the equivalent positions (equipoints). I have listed all 230 space groups and the symbols we use to enter them into the decoding program. Note that the combination of the given symmetry operations yields additional symmetry elements not listed separately (see the space group symmetry diagrams in the IT). For example, Fm3m is a cubic space group, and the 2-folds and 4-folds present are not listed in the symbol. Only the so called full symbol (F 4/m -3 2/m) shows these additional elements.
At this point it is worthwhile to recall that protein structures are restricted to non-mirror and non-inversion symmetry operations. This effectively reduces the number of possible groups to the 65 non-enantiogenic space groups. The by far most common space groups for proteins are P 212121 and P 21. An entropic model indicates that these space groups are the least restrictive to packing (rigid body degrees of freedom) and thus they can be created in a larger number of ways (Wukovitz and Yates, Nature Structural Biology 2 (12), 1062-1067, 1995).
In order to account for the whole unit cell content, we need to apply the symmetry and Bravais operations to the asymmetric unit, in our case the protein (or something such as a dimer, perhaps with a non-crystallographic symmetry relating the 2 monomers). The easiest way to see this at work is to use the following program to decode the space group and to read through the output. An explanation for the output is available.
Try a centrosymmetric space group. See what happens to the number of equivalent positions created.
Decode a space group with Bravais centering. What does it add to the equipoints created?
What is the maximum number of equivalent positions in a space group? (hint : try a higher symmetric space group such as F d 3 m).
Based on that number (and neglecting that such a space group is not chiral) would you expect a protein with such a unit cell?
FINAL QUIZ : go back to the introduction and look at the RGFP crystal. What is the spacegroup and its symmetry operator(s)? Click here to submit your answer. If your answer is right, you will be listed in the Space Group Hall of Fame. If not, I'll reply and explain. In case you worry, I will not list wrong answers.
![]() Real life example for
protein unit cell
Real life example for
protein unit cell
By now you should have a feel for space group symmetry and how to interpret a symbol. It is now time to revisit the diffraction experiment in detail and to dwell on the relations between the space group symmetry and the diffraction data. You may save a lot of time on data collection and will have less problems later in the structure determination by understanding these concepts. You can read more about crystal geometry and space groups in the first pages 1, 2, 3 of Chapter 5 of my book Biomolecular Crystallography or buy the book from Amazon.